
El experimento de los investigadores contó con paredes transparentes para permitir el acceso visual completo y utilizó una visualización de flujo de última generación. Crédito:Foto:Michael Schatz
Las turbulencias juegan un papel clave en nuestra vida diaria, provocando viajes en avión llenos de baches, afectando el tiempo y el clima, limitando la eficiencia de combustible de los automóviles que conducimos e impactando en las tecnologías de energía limpia. Sin embargo, los científicos e ingenieros se han preguntado cómo predecir y alterar los flujos de fluidos turbulentos, y durante mucho tiempo ha sido uno de los problemas más desafiantes de la ciencia y la ingeniería.
Ahora, los físicos del Instituto de Tecnología de Georgia han demostrado, numérica y experimentalmente, que la turbulencia se puede entender y cuantificar con la ayuda de un conjunto relativamente pequeño de soluciones especiales para las ecuaciones que gobiernan la dinámica de fluidos que se pueden precalcular para una geometría particular. de una vez por todas.
"Durante casi un siglo, la turbulencia se ha descrito estadísticamente como un proceso aleatorio", dijo Roman Grigoriev. "Nuestros resultados proporcionan la primera ilustración experimental de que, en escalas de tiempo adecuadamente cortas, la dinámica de la turbulencia es determinista y la conecta con las ecuaciones de gobierno deterministas subyacentes".
Los hallazgos se publicaron en Proceedings of the National Academy of Sciences el 19 de agosto de 2022. El equipo de investigadores estuvo dirigido por Grigoriev y Michael Schatz, profesores de la Escuela de Física de Georgia Tech que han colaborado en varios proyectos de investigación durante las últimas dos décadas.
Schatz y Grigoriev se unieron al estudio de los estudiantes graduados de la Facultad de Física Chris Crowley, Joshua Pughe-Sanford y Wesley Toler, junto con Michael Krygier, un científico postdoctoral en Sandia National Laboratories, quien desarrolló los solucionadores numéricos del estudio como estudiante graduado en Tecnología de Georgia.
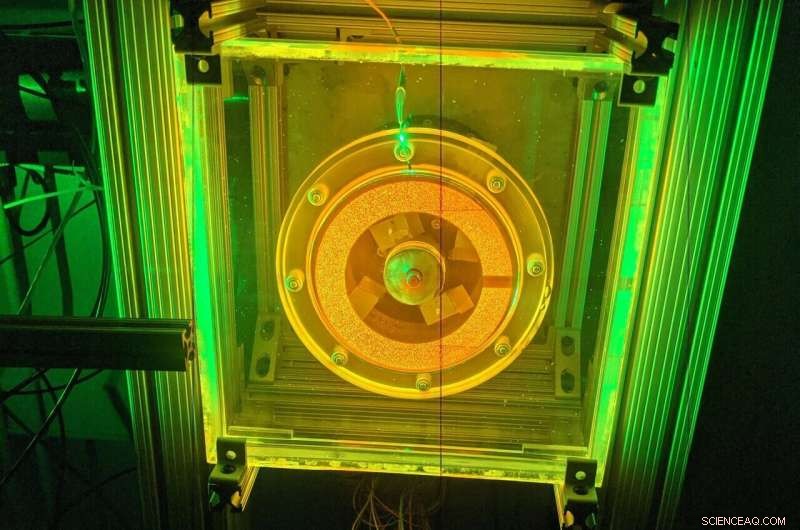
La configuración permitió a los investigadores reconstruir el flujo siguiendo el movimiento de millones de partículas fluorescentes suspendidas. Crédito:Foto:Michael Schatz
Una nueva 'hoja de ruta' para la investigación de turbulencias
Predecir cuantitativamente la evolución de los flujos turbulentos y, de hecho, casi cualquiera de sus propiedades, es bastante difícil. "La simulación numérica es el único enfoque de predicción existente confiable", dijo Grigoriev. "Pero puede ser terriblemente costoso. El objetivo de nuestra investigación era hacer que la predicción fuera menos costosa".
Los investigadores crearon una nueva "hoja de ruta" de la turbulencia al observar un flujo turbulento débil que estaba confinado entre dos cilindros que giraban independientemente, lo que le dio al equipo una forma única de comparar las observaciones experimentales con los flujos calculados numéricamente, debido a la ausencia de "efectos finales". que están presentes en geometrías más familiares, como el flujo por una tubería.
"La turbulencia se puede considerar como un automóvil que sigue una secuencia de caminos", dijo Grigoriev. "Quizás una analogía aún mejor es un tren, que no solo sigue una vía férrea en un horario prescrito, sino que también tiene la misma forma que la vía férrea que sigue".
El experimento contó con paredes transparentes para permitir el acceso visual completo y utilizó una visualización de flujo de última generación para permitir a los investigadores reconstruir el flujo mediante el seguimiento del movimiento de millones de partículas fluorescentes suspendidas. In parallel, advanced numerical methods were used to compute recurrent solutions of the partial differential equation (Navier-Stokes equation), governing fluid flows under conditions exactly matching experiment.
It is well-known that turbulent fluid flows exhibit a repertoire of patterns—referred to as "coherent structures" in the field—that have a well-defined spatial profile but appear and disappear in an apparently random manner. By analyzing their experimental and numerical data, the researchers discovered that these flow patterns and their evolution resemble those described by the special solutions they computed. These special solutions are both recurrent and unstable, meaning they describe repeating flow patterns over short intervals of time. Turbulence tracks one such solution after another, which explains what patterns can appear, and in what order.
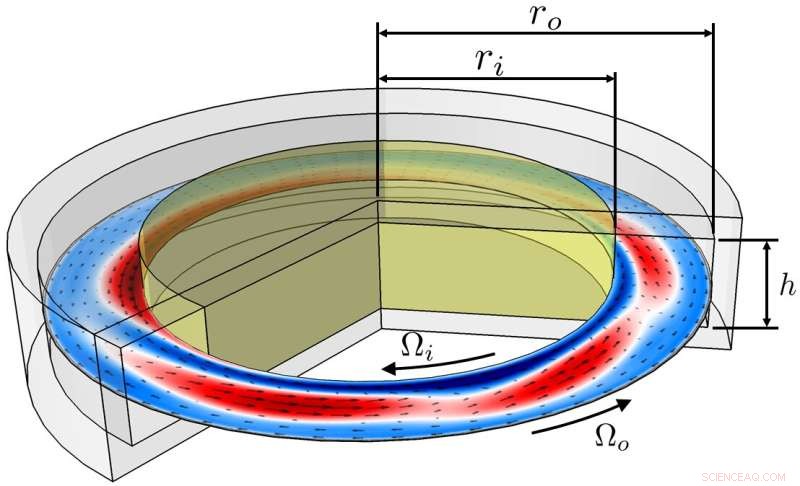
A schematic of the physicists' research. Credit:Michael Schatz, Roman Grigoriev.
Recurrent solutions, two frequencies
"All the recurrent solutions that we found in this geometry turned out to be quasi-periodic—that is, characterized by two different frequencies," said Grigoriev. One frequency described the overall rotation of the flow pattern around the axis of symmetry of the flow, while the other described the changes in the shape of the flow pattern in a reference frame co-rotating with the pattern. The corresponding flows repeat periodically in these co-rotating frames.
"We then compared turbulent flows in experiment and direct numerical simulations with these recurrent solutions and found turbulence to closely follow (track) one recurrent solution after another, for as long as turbulent flow persisted," Grigoriev said. "Such qualitative behaviors were predicted for low-dimensional chaotic systems, such as the famous Lorenz model, derived six decades ago as a greatly simplified model of the atmosphere."
The work represents the first experimental observation of chaotic motion tracking recurrent solutions actually observed in turbulent flows. "The dynamics of turbulent flows are, of course, far more complicated due to the quasi-periodic nature of recurrent solutions," Grigoriev added.
"Using this method, we conclusively showed that the organization of turbulence in both space and time is well captured by these structures," the researchers said. "These results lay the foundation for representing turbulence in terms of coherent structures and leveraging their persistence in time to overcome the devastating effects of chaos on our ability to predict, control, and engineer fluid flows."
A new dynamical foundation for 3D fluid flows
These findings most immediately impact the community of physicists, mathematicians, and engineers who are still trying to understand fluid turbulence, which remains "perhaps the greatest unsolved problem in all of science," Grigoriev said.
"This work builds and expands on previous work on fluid turbulence by the same group, some of which was reported at Georgia Tech in 2017," he added. "Unlike the work discussed in that publication, which focused on idealized two-dimensional fluid flows, present research addresses the practically important and more complicated three-dimensional flows."
Ultimately, the team's study lays a mathematical foundation for fluid turbulence which is dynamical, rather than statistical, in nature—and hence has the capability to make quantitative predictions, which are crucial for a variety of applications.
"It can give us the ability to dramatically improve the accuracy of weather forecasts and, most notably, enable prediction of extreme events such as hurricanes and tornadoes," said Grigoriev. "Dynamical framework is also essential for our ability to engineer flows with desired properties, for instance, reduced drag around vehicles to improve fuel efficiency, or enhanced mass transport to help remove more carbon dioxide from the atmosphere in the emerging direct air capture industry." Researchers reveal multi-scale characteristics of helicity in wall-bounded turbulent flows